分類:燒腦題:修訂版本之間的差異
出自六年制學程
(→中學數學題) |
|||
| (未顯示同用戶所作出之49次版本) | |||
| 第 24 行: | 第 24 行: | ||
'''圓相關'''<br/> | '''圓相關'''<br/> | ||
<img src='http://jendo.org/files/解數學題/圓內四個方形求面積.jpg' width=400 height=* />  | <img src='http://jendo.org/files/解數學題/圓內四個方形求面積.jpg' width=400 height=* />  | ||
| − | <img src='http://jendo.org/files/解數學題/ | + | <details><summary><img src='http://jendo.org/files/解數學題/方內圓用邊角矩形求半徑.png' width=400 height=* /></summary> |
| − | <img src='http://jendo.org/files/解數學題/三圓求陰影面積.jpg' width=400 height=* /> | + | 圖半徑 R ,R-1.R-2,R 構成直角三角形<br/> |
| + | 解出三邊為 3.4.5 | ||
| + | </details> | ||
| + | <details><summary><img src='http://jendo.org/files/解數學題/三圓求陰影面積.jpg' width=400 height=* /></summary> | ||
| + | 兩圖相切,連心線過切點。左側中圖半徑 2 ,右側中圖半徑 r 。<br/> | ||
| + | 2,(4-r),(2+r) 構成直角三角形,解出 r 為 4/3 。 | ||
| + | </details> | ||
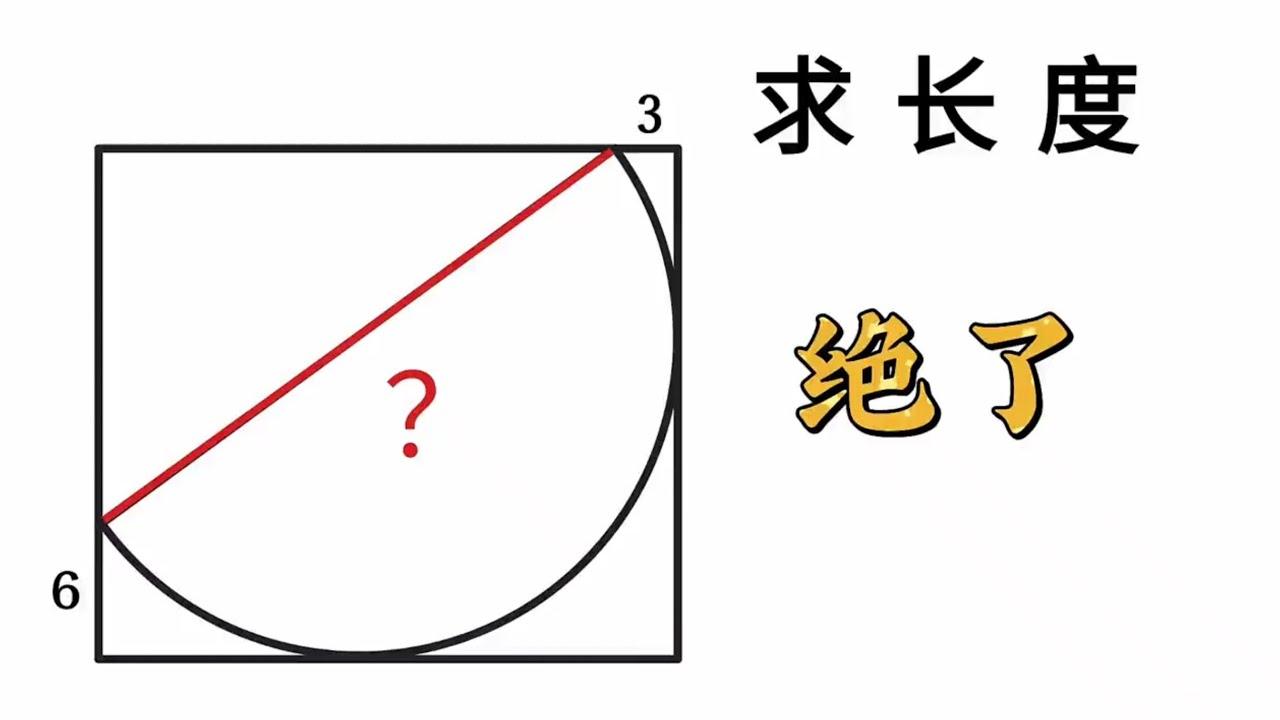
<img src='http://jendo.org/files/解數學題/用圓內四邊形求半徑.jpg' width=400 height=* /><br/> | <img src='http://jendo.org/files/解數學題/用圓內四邊形求半徑.jpg' width=400 height=* /><br/> | ||
| + | <details><summary><img src='http://jendo.org/files/解數學題/矩形內半圓1.jpg' width=400 height=* /></summary><img src='http://jendo.org/files/解數學題/矩形內半圓2.jpg' width=400 height=* /></details> | ||
'''三角形相關'''<br/> | '''三角形相關'''<br/> | ||
| − | <img src='http://jendo.org/files/解數學題/直角三角兩邊生正方形求陰影面積.jpg' width=400 height=* /> | + | <details><summary><img src='http://jendo.org/files/解數學題/直角三角兩邊生正方形求陰影面積.jpg' width=400 height=* /></summary> |
| + | 陰影三角形順時鐘轉 90° ,與 3,4,5 三角形同底等高。面積為 6 。 | ||
| + | </details><br/> | ||
<img src='http://jendo.org/files/解數學題/已知三邊求頂角.jpg' width=400 height=* /><br/> | <img src='http://jendo.org/files/解數學題/已知三邊求頂角.jpg' width=400 height=* /><br/> | ||
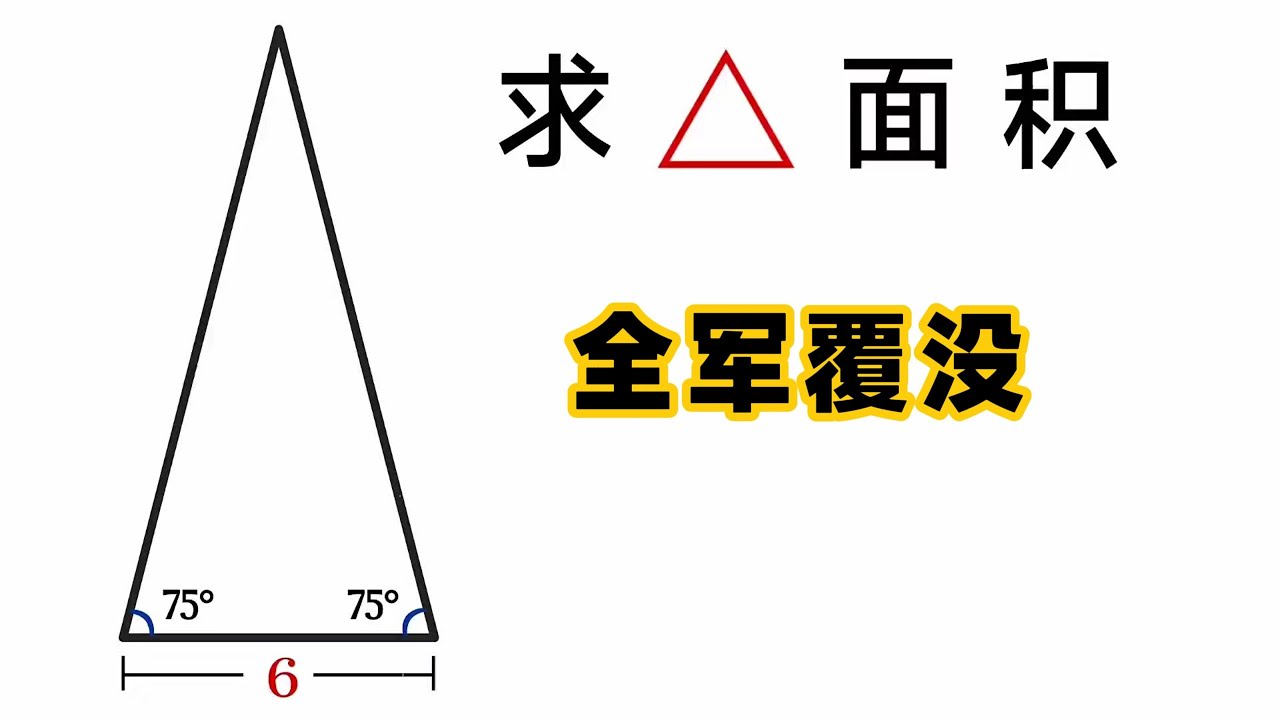
| − | <img src='http://jendo.org/files/解數學題/75度三角形求面積.jpg' width=400 height=* /> | + | <details><summary><img src='http://jendo.org/files/解數學題/部分角部分邊1.jpg' width=400 height=* /></summary><img src='http://jendo.org/files/解數學題/部分角部分邊2.jpg' width=400 height=* /></details> |
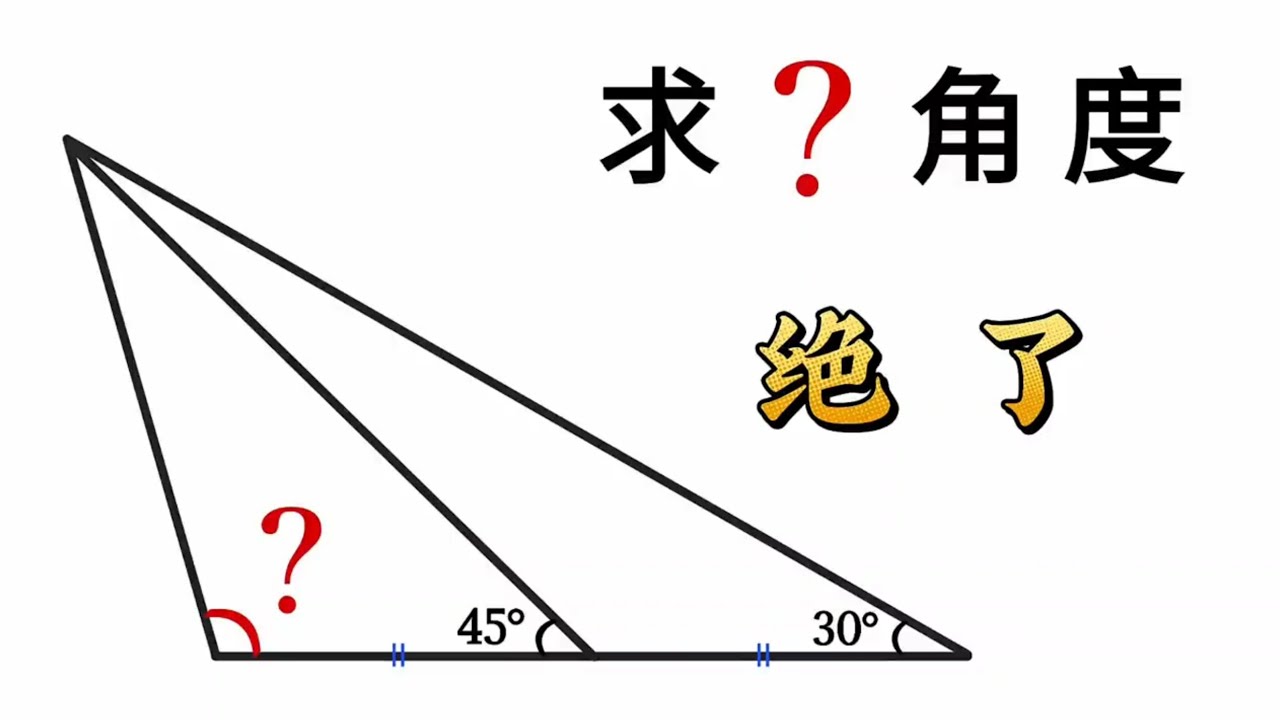
| − | <img src='http://jendo.org/files/解數學題/30_45_75度角.jpg' width=400 height=* /><br/> | + | <div style='float:right'><img src='https://upload.wikimedia.org/wikipedia/commons/a/a2/Diagram_showing_steps_towards_deriving_half-angle_formula_for_cosine.svg' width=400 height=* /></div> |
| + | '''半角公式相關'''<br/> | ||
| + | :'''cosθ = 2cos<sup>2</sup>(θ/2) - 1''' 之圖形證明 | ||
| + | :如右圖: | ||
| + | # 作土黃色三角形(斜邊為<span style='text-decoration:overline'>BD</span>)與粉紅色三角形(斜邊為<span style='text-decoration:overline'>AB</span>)翻轉對稱。 | ||
| + | # 作藍色 △ADE 與粉紅色三角形(斜邊為<span style='text-decoration:overline'>AB</span>)相似, ∠DEF 為直角 | ||
| + | # ∵內錯角相等 ∴ <span style='text-decoration:overline'>BD</span> 平行 <span style='text-decoration:overline'>AE</span> | ||
| + | #橘色斜邊 <span style='text-decoration:overline'>BD</span> 為 1 ,其矩形對邊 <span style='text-decoration:overline'>FE</span> 也為 1 。 | ||
| + | # ∵<span style='text-decoration:overline'>AB</span> 為 1 ∴ <span style='text-decoration:overline'>AF</span> 為 COSθ (看紫色邊三角形)。 | ||
| + | # ∵粉紅色三角形(斜邊為<span style='text-decoration:overline'>AB</span>)與 △ADE 相似 ∴ <span style='text-decoration:overline'>AE</span> = <span style='text-decoration:overline'>AD</span> × COS(θ/2) = 2COS<sup>2</sup>(θ/2) | ||
| + | # ∵<span style='text-decoration:overline'>AF</span> = <span style='text-decoration:overline'>AE</span> - <span style='text-decoration:overline'>FE</span> ∴ cosθ = 2cos<sup>2</sup>(θ/2) - 1 | ||
| + | |||
| + | <details><summary><img src='http://jendo.org/files/解數學題/75度三角形求面積.jpg' width=400 height=* /></summary><img src='https://upload.wikimedia.org/wikipedia/commons/1/14/15°75°90°triangle_sides.png' width=400 height=* /><br/>1:(2+√<span style='text-decoration:overline'>3</span>)=(2-√<span style='text-decoration:overline'>3</span>):1</details> | ||
| + | <details><summary><img src='http://jendo.org/files/解數學題/30_45_75度角.jpg' width=400 height=* /></summary> | ||
| + | 左側隱藏直角三角形之高為 1 ,兩個等長的底為 x ,左側隱藏直角三角形之底為(1-(√<span style='text-decoration:overline'>3</span>-1))=2-√<span style='text-decoration:overline'>3</span><br/>左側隱藏三角形為15°75°90°之直角三角形。 | ||
| + | </details> | ||
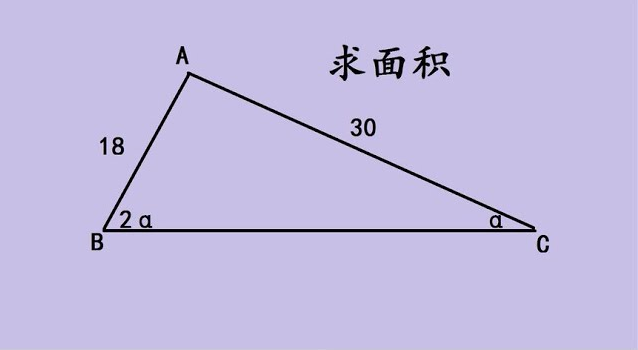
| + | <details><summary><img src='http://jendo.org/files/解數學題/兩邊兩底角.png' width=400 height=* /></summary> | ||
| + | 右側邊(18)垂直翻轉 180° 即成為典型的半角公式圖形。<br/> | ||
| + | <img src='https://upload.wikimedia.org/wikipedia/commons/9/9b/Half-angle_formula_side_length.svg' width=400 height=* /><br/> | ||
| + | a 以 18 代入, b 以 30 代入,得出高為 5√<span style='text-decoration:overline'>11</span> , 18 為斜邊的直角三角形底為 7 ,大三角形底 32 ,面積 80√<span style='text-decoration:overline'>11</span> 。 | ||
| + | </details> | ||
| + | <details><summary>①tanA=1 ,求 tan2A ?</summary> | ||
| + | tan2A=2tanA/(1-tan<sup>2</sup>A)=∞ , ∠A=45° ,2A=90° 。 | ||
| + | </details> | ||
| + | <img src='http://jendo.org/files/解數學題/values-of-sin-18-cos-18-sin-36-cos-36-sin-54-cos-54.png' width=400 height=* />[https://physicscatalyst.com/article/values-of-sin-18-cos-18-cos-72-sin-36-cos-36-sin-54/ 公式解說(英文)]<br/> | ||
| + | <img src='http://jendo.org/files/解數學題/value-15°-to-75°.png' width=400 height=* /><br/> | ||
| + | <img src='http://jendo.org/files/解數學題/value-18°-to-72°.png' width=400 height=* /><br/> | ||
| + | <img src='http://jendo.org/files/解數學題/value-18°-to-72°-sin-cos.png' width=400 height=* /><br/> | ||
| + | ②tan36°≈ 0.726543 => tan72°≈ 3.077684 <br/> | ||
| + | <details><summary>③tanA=1/√<span style='text-decoration:overline'>3</span>(≈0.57735) ,求 tan2A ?</summary> | ||
| + | tan2A=2tanA/(1-tan<sup>2</sup>A)=√<span style='text-decoration:overline'>3</span>(≈1.732), ∠A=30° ,2A=60° 。 | ||
| + | </details> | ||
| + | <details><summary>④tanA=1/2 ,求 tan2A ?</summary> | ||
| + | tan2A=2tanA/(1-tan<sup>2</sup>A)=4/3 ,此為 3,4,5 三角形 。 ∠A=26.5° , 2A 對 4 ,約 53.13° 。<br/> | ||
| + | <img src='https://upload.wikimedia.org/wikipedia/commons/9/9e/Half-angle_formula_side_length_3_4_5.svg' width=400 height=* /> | ||
| + | </details> | ||
| + | ⑤tan22.5°= 1/(1+√<span style='text-decoration:overline'>2</span>) ≈ 0.41421 => tan45°= 1<br/> | ||
| + | <details><summary>⑥<img src='http://jendo.org/files/解數學題/tan_2a.png' width=400 height=* /></summary> | ||
| + | tan2A=2tanA/(1-tan<sup>2</sup>A)=3/4 ,此為 3,4,5 三角形 。 ∠A=18.4° , 2A 對 3 ,約 36.87° 。<br/> | ||
| + | <img src='https://upload.wikimedia.org/wikipedia/commons/9/9b/Half-angle_formula_side_length_4_3_5.svg' width=400 height=* /> | ||
| + | </details> | ||
| + | ⑦tan18°≈ 0.32492 => tan36°≈ 0.726543 <br/> | ||
| + | <details><summary>⑧tanA=1/(2+√<span style='text-decoration:overline'>3</span>)≈0.267949 ,求 tan2A ?</summary> | ||
| + | tan2A=2tanA/(1-tan<sup>2</sup>A)=1/√<span style='text-decoration:overline'>3</span>(≈0.57735), ∠A=15° ,2A=30° 。<br/> | ||
| + | <img src='https://upload.wikimedia.org/wikipedia/commons/1/14/15°75°90°triangle_sides.png' width=400 height=* /> | ||
| + | </details> | ||
| + | <table class=nicetable> | ||
| + | <tr><th>角度</th><th>tanA</th><th>tan2A</th></tr> | ||
| + | <tr><td>①45°</td><th>1</th><th>∞</th></tr> | ||
| + | <tr><td>②36°</td><th>0.726543</th><th>3.077684</th></tr> | ||
| + | <tr><td>③30°</td><th>0.57735</th><th>1.732</th></tr> | ||
| + | <tr><td>④26.5°</td><th>0.5</th><th>4/3</th></tr> | ||
| + | <tr><td>⑤22.5°</td><th>0.41421</th><th>1</th></tr> | ||
| + | <tr><td>⑥18.4°</td><th>1/3</th><th>3/4</th></tr> | ||
| + | <tr><td>⑦18°</td><th>0.32492</th><th>0.726543</th></tr> | ||
| + | <tr><td>⑧15°</td><th>0.267949</th><th>0.57735</th></tr> | ||
| + | <tr><td>⑨0°</td><th>0</th><th>0</th></tr> | ||
| + | </table> | ||
'''因式分解:''' | '''因式分解:''' | ||
a<sup>5</sup>+a<sup>4</sup>+1 | a<sup>5</sup>+a<sup>4</sup>+1 | ||
2025年2月2日 (日) 08:48的最新修訂版本
求 a b
a2+b2=20 ,且 b 是 a 的小數部分。
|b|<1 且 b2 < 1
a2 > 19
設 a=n+b n為整數,且必為 ±4
(±4+b)2+b2=20
16±8b+2b2=20 => b2±4b-2=0
b=(∓4±√16+8)/2
b=∓2±√6
|b|<1 ,者 僅有 √6-2 , 2-√6
a=4+b=√6+2 , -(√6+2)
中學數學題
圓相關
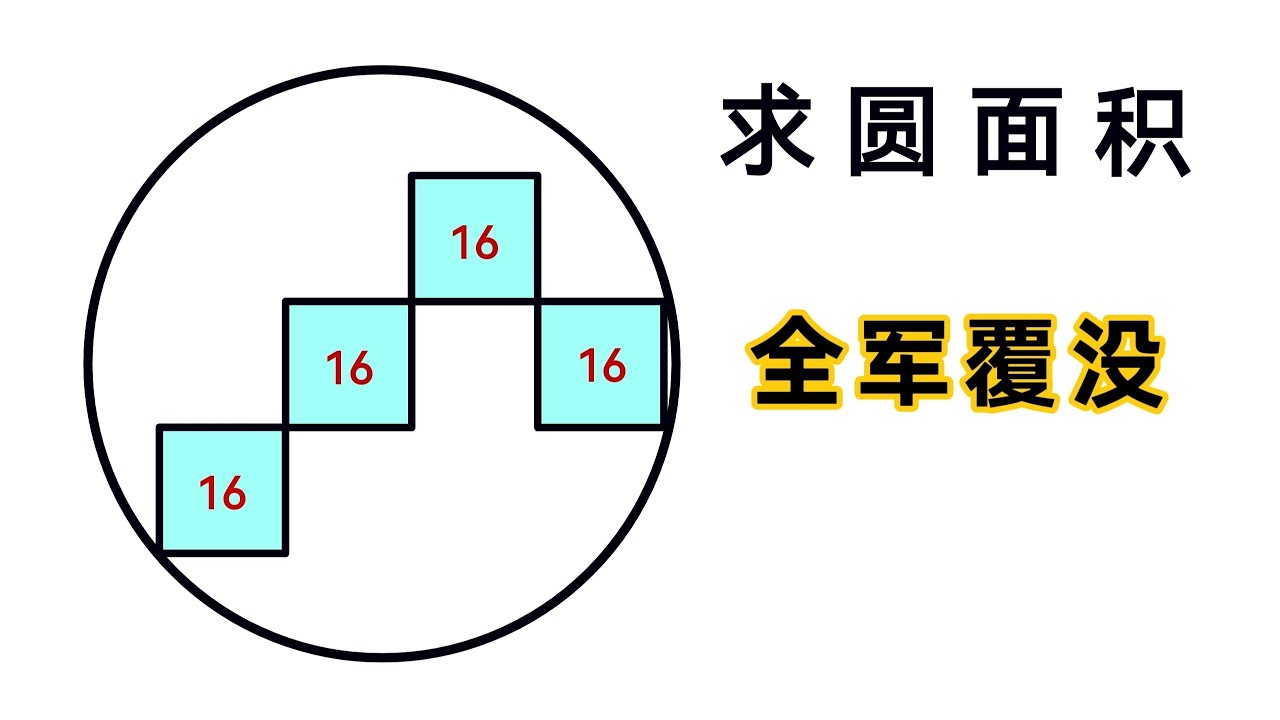
解出三邊為 3.4.5
2,(4-r),(2+r) 構成直角三角形,解出 r 為 4/3 。
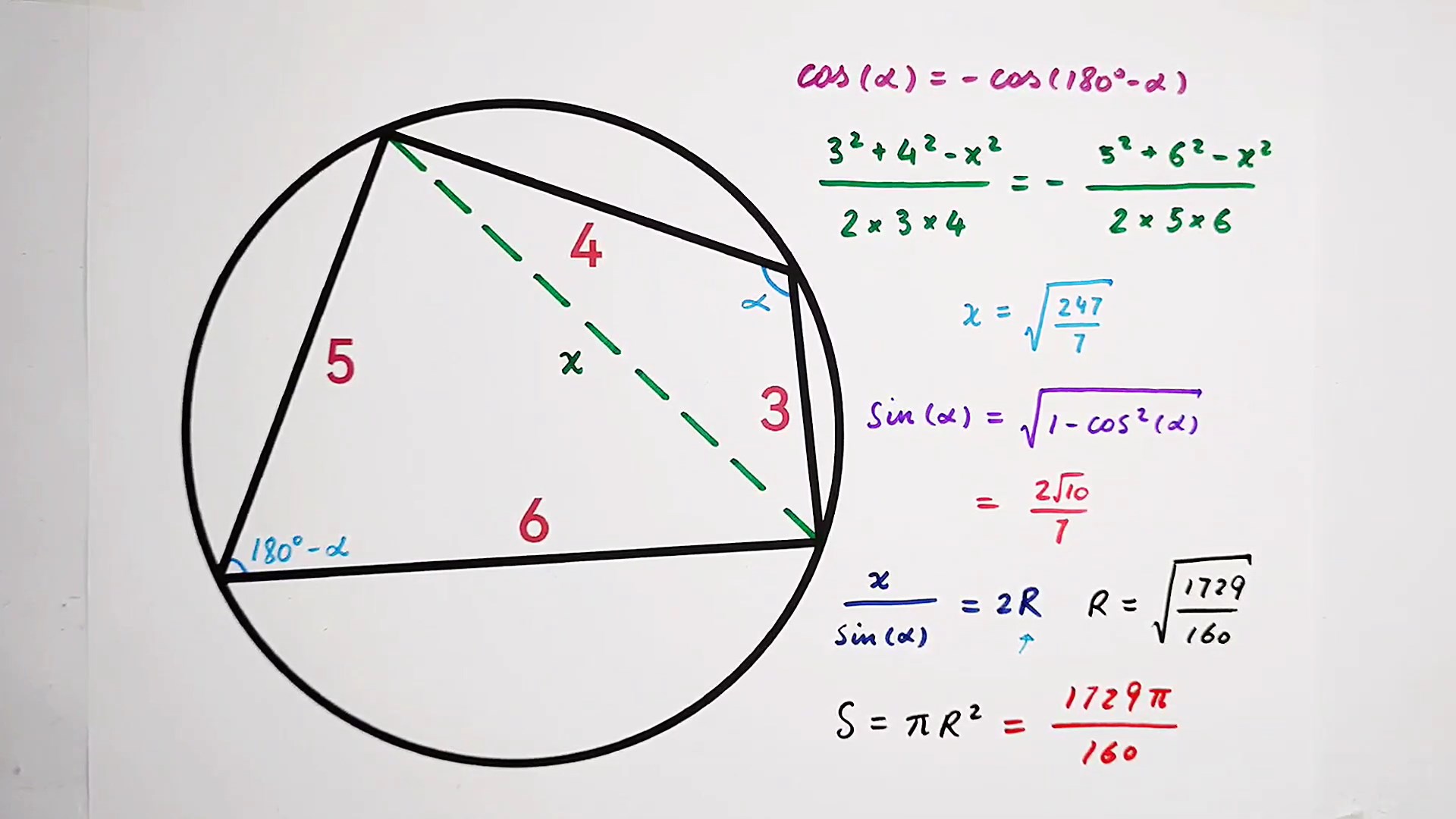

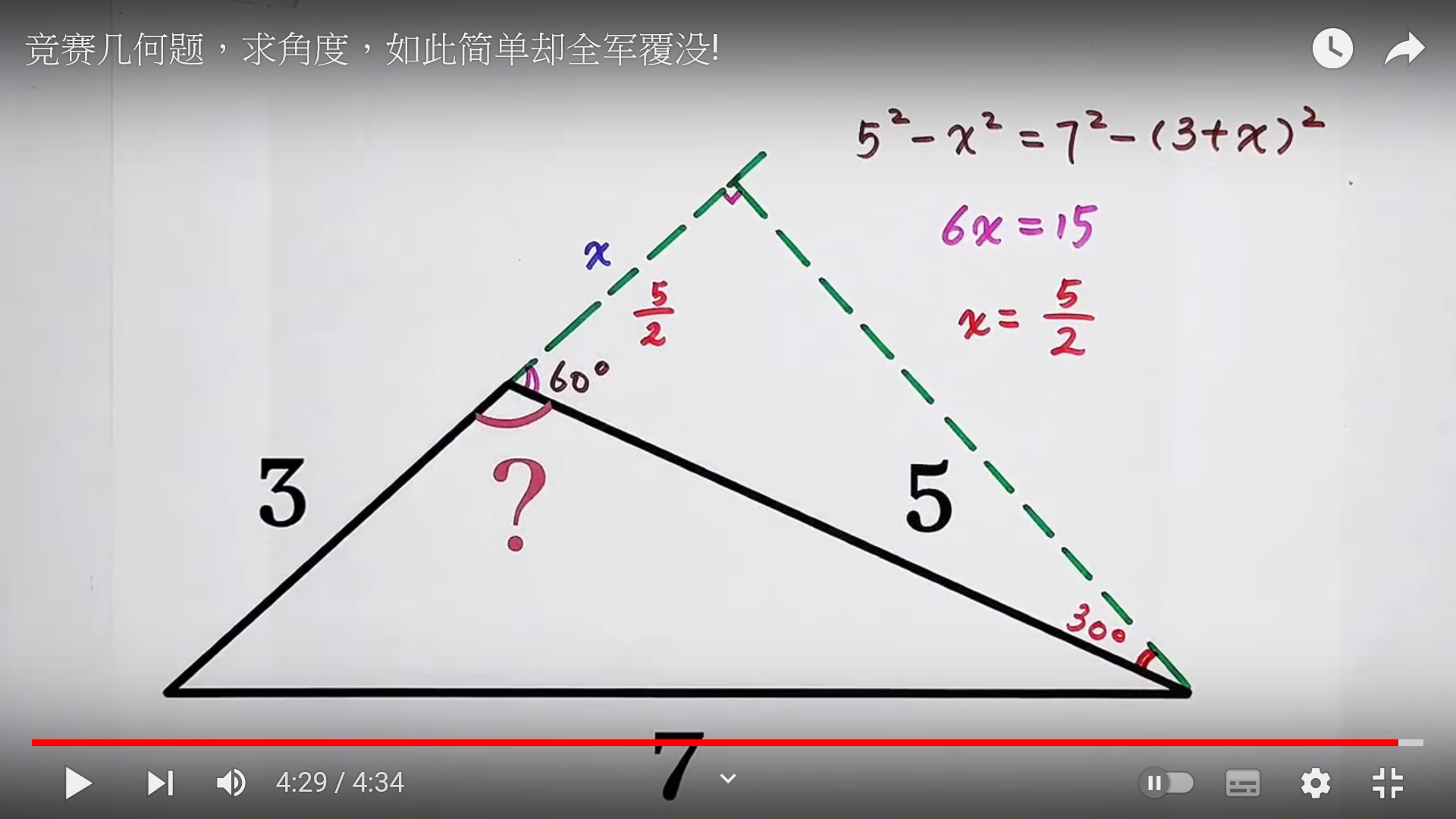
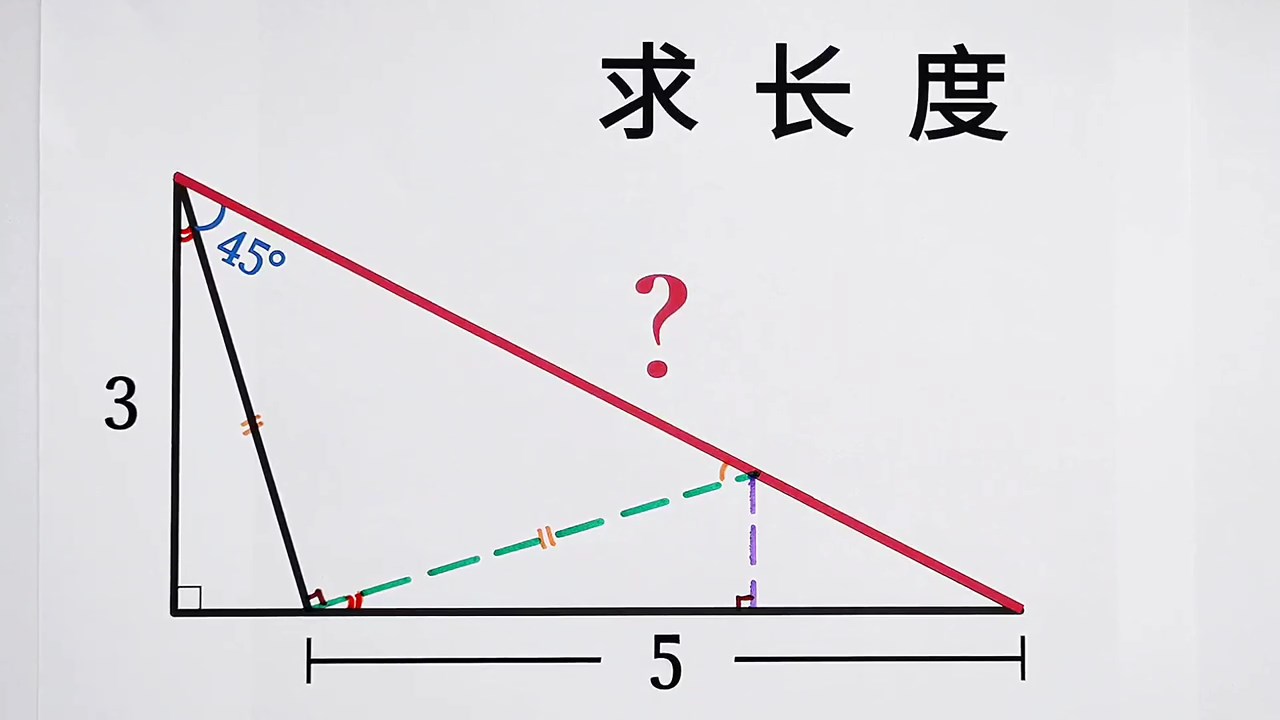
半角公式相關
- cosθ = 2cos2(θ/2) - 1 之圖形證明
- 如右圖:
- 作土黃色三角形(斜邊為BD)與粉紅色三角形(斜邊為AB)翻轉對稱。
- 作藍色 △ADE 與粉紅色三角形(斜邊為AB)相似, ∠DEF 為直角
- ∵內錯角相等 ∴ BD 平行 AE
- 橘色斜邊 BD 為 1 ,其矩形對邊 FE 也為 1 。
- ∵AB 為 1 ∴ AF 為 COSθ (看紫色邊三角形)。
- ∵粉紅色三角形(斜邊為AB)與 △ADE 相似 ∴ AE = AD × COS(θ/2) = 2COS2(θ/2)
- ∵AF = AE - FE ∴ cosθ = 2cos2(θ/2) - 1

1:(2+√3)=(2-√3):1
左側隱藏三角形為15°75°90°之直角三角形。
a 以 18 代入, b 以 30 代入,得出高為 5√11 , 18 為斜邊的直角三角形底為 7 ,大三角形底 32 ,面積 80√11 。
①tanA=1 ,求 tan2A ?
tan2A=2tanA/(1-tan2A)=∞ , ∠A=45° ,2A=90° 。 公式解說(英文)
公式解說(英文)


②tan36°≈ 0.726543 => tan72°≈ 3.077684
③tanA=1/√3(≈0.57735) ,求 tan2A ?
tan2A=2tanA/(1-tan2A)=√3(≈1.732), ∠A=30° ,2A=60° 。④tanA=1/2 ,求 tan2A ?
tan2A=2tanA/(1-tan2A)=4/3 ,此為 3,4,5 三角形 。 ∠A=26.5° , 2A 對 4 ,約 53.13° 。⑥
tan2A=2tanA/(1-tan2A)=3/4 ,此為 3,4,5 三角形 。 ∠A=18.4° , 2A 對 3 ,約 36.87° 。⑧tanA=1/(2+√3)≈0.267949 ,求 tan2A ?
tan2A=2tanA/(1-tan2A)=1/√3(≈0.57735), ∠A=15° ,2A=30° 。
| 角度 | tanA | tan2A |
|---|---|---|
| ①45° | 1 | ∞ |
| ②36° | 0.726543 | 3.077684 |
| ③30° | 0.57735 | 1.732 |
| ④26.5° | 0.5 | 4/3 |
| ⑤22.5° | 0.41421 | 1 |
| ⑥18.4° | 1/3 | 3/4 |
| ⑦18° | 0.32492 | 0.726543 |
| ⑧15° | 0.267949 | 0.57735 |
| ⑨0° | 0 | 0 |
因式分解: a5+a4+1
此分類目前未包含頁面或媒體。